In our example, Force = ( 80 psi ) x (3.14 square inches) = 251 pounds

Click to Enlarge

Notice the difference between force and pressure. Each square inch of piston area feels a force in pounds equal to the pressure of 80 pounds per square inch. The total area of the face of the piston exposed to the air is 3.14 square inches. So, the total force exerted on the piston is 251 pounds.

2. Pressure at a certain depth in water

Scuba divers know that as you go down to greater depths, the water pressure increases. In fact, the increase in pressure is 14.7 psi for every 34 feet of additional depth. A diver that descends to a depth of 100 feet must withstand a pressure of ...

( 100 ft / 34 ) x 14.7 = 43.24 psi

This pressure is in addition to the normal atmospheric pressure at the surface. The pressure limits the depth to which unprotected divers can go, and the pressure causes lots of problems.

The point is that as you descend deeper into a fluid, whether it be water or air or whatever, the pressure increases. And if you go upward toward the surface, the pressure becomes less.

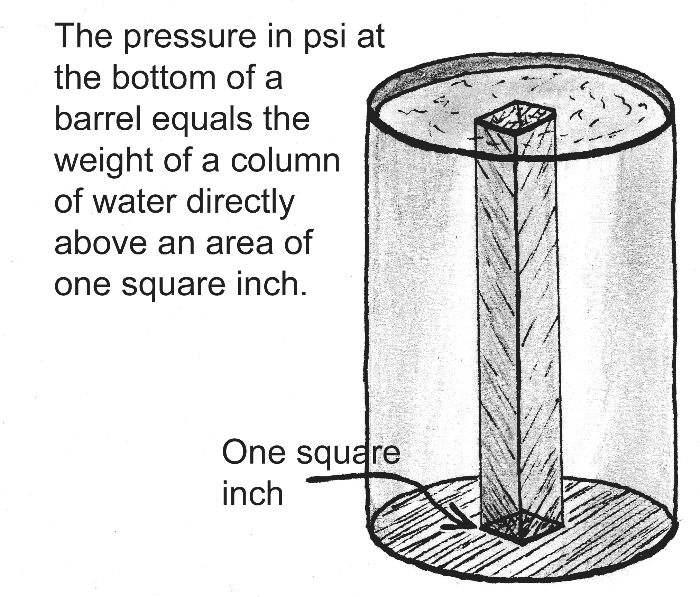
Here is another view of the pressure at the bottom of a tank of water. Suppose the tank is rather tall so that the depth of water in the tank is 10 feet. What will be the pressure at the bottom of the tank?

We can use the relationship above to find the answer:

( 10 ft / 34 ) x 14.7 = 4.32 psi

This means that each square inch of surface area on the bottom of the tank is being pushed downward with a force of 4.32 pounds.

Now consider this: suppose we have a tall, skinny column of water 10 feet tall, with the column being one inch on each side. That is, its bottom surface area is 1 square inch. How much would the column of water weight? Sparing you the details of the math, the weight of the column of water would be 4.32 pounds. Here's the point: the pressure at the bottom of the tank in psi is the same as the weight of the water supported by each square inch of the bottom surface of the tank.

Click to Enlarge

3. Atmospheric pressure

We live at the bottom of the atmosphere of the earth. And, just as the creatures living at the bottom of the ocean are subjected to the pressure exerted on them by the water, we are subjected to the pressure exerted on us by the atmosphere.

Air is heavy. It is a lot heavier than most people realize. One cubic yard of air at sea level pressure and at a temperature of 70 F weighs almost exactly 2 pounds. The air in a room 12 ft x 14 ft with an 8-foot ceiling weighs almost exactly 100 pounds.

To come to the point quickly, the average air pressure at sea level is about 14.7 psi. It varies from day to day, but this is the average pressure in pounds per square inch, in round numbers.

Suppose you go outside to an open area of a sidewalk and sketch a surface area of one square inch on the concrete. Draw a tiny square, one inch on a side. Then imagine the column of air directly above this little square, extending from the sidewalk all the way to outer space. How much would the air in this tall, skinny column weigh?

Remember the water in the tank above? Each square inch of bottom surface has to support the water above it. Likewise, each square inch of sidewalk has to support the column of air above it. Therefore, the column of air will weigh 14.7 pounds, the same as the pressure.

You may have thought, because air is rather heavy, that the column extending from the sidewalk all the way to outer space would weigh more than this. But it doesn't. Why?

Truth is, our atmosphere is rather thin; it is not very deep at all compared to the overall diameter of the earth. An analogy that is not too far off is that the atmosphere is about as thick as the skin of an onion, relatively speaking. However, the exact thickness of the atmosphere is hard to specify because the air just gradually thins out as we go higher. This confuses our onion-skin analogy just a bit.

4. Measuring air pressure with a column of liquid; the Barometer

One of the first methods used to measure pressure utilized a glass tube in the shape of a U that was partially filled with a liquid to form what we now know as a U-tube manometer. The pressure to be measured is connected to one arm of the U while the other is left open to the atmosphere. The pressure displaces the liquid by pushing down on the pressure side, forcing the liquid level to rise on the other side. The difference is heights of the liquid levels is proportional to the pressure being measured.

The pressure is specified by simply giving the distance between the two liquid levels. If water is used as the liquid, the pressure is given as so many inches of water. As you can imagine from a practical standpoint, only very low pressures can be measured in this manner.

Any convenient liquid can be used, but a U with shorter arms will result if the liquid is heavy. Accordingly, mercury is the liquid of choice because the density of mercury is something like 13.6 times as much as the density of water. This means that for arms of a given length, pressures 13.6 times as great can be measured if mercury is used. And if mercury is used, the pressure is given as so many inches of mercury. This is often written as in/Hg. (Chemical symbol for mercury is Hg.)

Gauge pressure vs. Absolute pressure

Click to Enlarge

Leaving one side of the U-tube open results in the pressure being measured relative to atmospheric pressure. This is because atmospheric pressure pushes down on the liquid in the open tube. A pressure measured relative to atmospheric is called a gauge pressure (psig). Indeed, most common pressure gauges indicate pressure relative to that of the atmosphere and therefore indicate gauge pressure.

Now suppose we connect a vacuum pump to the open arm of the U, pump out the air, and then seal off the end of the tube. The result is that no pressure will exist above the liquid in that side of the tube. It's a vacuum. (This ignores the vapor pressure of the liquid.) Pressures obtained with one arm evacuated and sealed off are absolute pressures (psia). The relationship between gauge and absolute pressure is Absolute pressure = Gauge pressure + Atmospheric pressure

Click to Enlarge

A Barometer

You probably realize that as we use a vacuum pump to remove the air from one side of the U tube, the liquid is going to shift away from the open tube and toward the pump. This is due to atmospheric pressure in the open tube pushing down on the liquid on that side and forcing it upward on the other.

Click to Enlarge

Further, the liquid will continue to rise toward the pump until the atmospheric pressure simply cannot push it up any more. The actual height to which it will go depends upon the liquid being used. For mercury, the liquid will rise until the upper level is about 30 inches above the lower level. We have invented a barometer. Furthermore, it will be a good one, assuming our vacuum pump is able to remove "all" the air from the tube that eventually gets sealed off. It may be a bit cumbersome to use, but it will be accurate. The reason it's a barometer is because the difference in height between the two columns is proportional to the atmospheric pressure. As the pressure goes up, the difference in height will increase. The reading of this barometer will be in inches of mercury, and will be very nearly 30 in/Hg.

Why doesn't the liquid get sucked all the way into the pump?

This is a good question. In fact, if the arms of the U-tube are too short for the liquid being used, this very thing can happen. And it will ruin your day because the liquid will contamimate the pump.

The thing that causes the liquid to shift is the atmospheric pressure on the open side. It is NOT the "suction" of the pump pulling upward on the liquid. In fact, there is no such thing as a suction. But, low pressure at one point acting in concert with a higher pressure somewhere else gives the appearance of a suction. The key idea is that of "pressure differential."

The word "suction" implies a pulling effect. However, you can't pull on a material such as air or water. It's a little bit like pushing on a rope! So, suction is not a real, physical thing.

Atmospheric pressure is just so much and no more. It's about 14.7 psi, in fact, and this corresponds roughly to about 30 in/Hg. So, if mercury is the liquid in the tube, it will shift until the upper level is about 30 inches above the lower level, and then it will stabilize. You can let the pump run for hours or days and the level will go no higher.

Water barometer

Water can be used as the liquid to make a pretty good barometer, but there is a practical difficulty in that the arms of the U tube need to be about 34 feet long. That is, atmospheric pressure is able to push a column of water upward a distance of 34 feet above the level at which the pressure is applied.

The first barometer was developed by Evangelista Torricelli in 1643, in the era of Issac Newton. One of his earlier models used water as the liquid. Story has it that he mounted the thing (34 feet tall) in the village square so the townspeople could see it. But they made him take it down because every time the liquid dropped down, the weather turned bad. And they didn't need any more bad weather.

After Mr. Torricelli had developed a workable and portable mercury barometer, he and his friends read the thing very carefully while down in the valley, and then carried it up to the top of a nearby mountain. Ahh ha! The barometer indicated a lower pressure at the top of the mountain than it did down in the valley. This gave the first indication that the atmosphere of the earth had a finite depth rather than extending upward forever.

Now, at the beginning of this article, we said that for every 34 feet a diver goes down into water, the pressure increases by 14.7 psi. Do you see the relationship? That is the equivalent of atmospheric pressure and the height of the corresponding water column:

Atmospheric pressure = 14.7 psi = 30 in/Hg = 34 feet of water

All these numbers are approximate, of course.

Aneroid barometers

Most barometers we encounter use a mechanical mechanism of some sort rather than columns of liquid to sense atmospheric pressure. These are called aneroid barometers. The word "aneroid" somehow means "without liquid."

A typical aneroid barometer utilizes a bellows made of thin metal that expands or contracts as the air pressure changes. The movement of the bellows is communicated to the indicator needle by an intricate linkage that also provides for adjustment and calibration. In fact, the altimeters on our planes are aneroid barometers with a few modifications.

5. Variation of air pressure with altitude

Just as a diver experiences a decrease in pressure as he or she rises toward the surface, we experience a similar decrease in pressure as we climb to higher altitudes in our planes, or climb to the top of a mountain, or go from the basement to upstairs at home. (A sensitive altimeter will actually respond to a one-story change in elevation.)

Atmospheric pressure (same as barometric pressure) varies somewhat on a day-to-day basis. When the readings are taken over a long period of time in many different locations and then averaged, it is found that the average pressure at sea level is very nearly 76 centimeters (cm) of mercury. This value has been taken (somewhat arbitrarily) as the "standard value."

There are 2.54 centimeters in one inch. Now, divide 76 cm by 2.54 to convert to inches. What do you get? Answer: 29.921 inches of mercury. This is the origin of the 29.92 in/Hg standard sea-level barometric pressure.

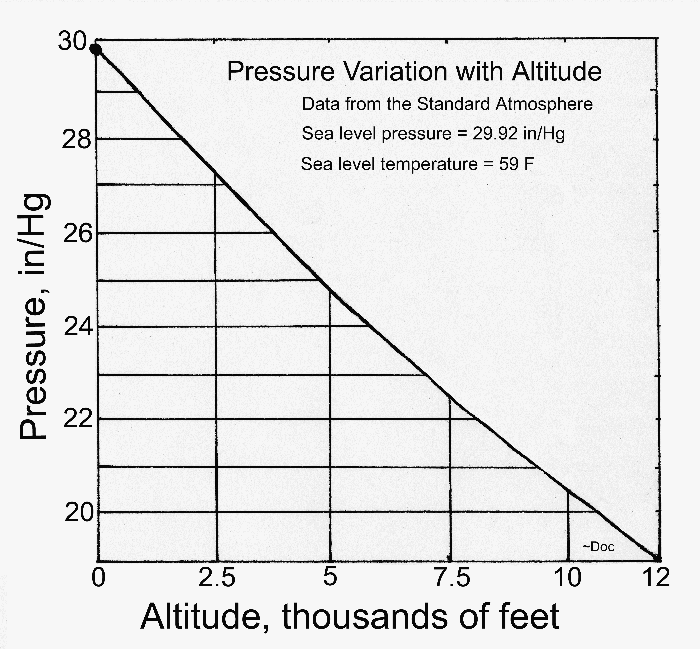
The standard atmosphere

In scientific circles, a "standard atmosphere" has been defined that specifies the temperature, pressure, and density of the air from sea level to an altitude of over 50 miles. The base line of this standard is taken as 29.92 in/Hg at sea level at a temperature of 59 F.

A standard atmosphere calculator with graphs and other information can be found at http://www.digitaldutch.com/atmoscalc/

The calculator lets you insert an altitude, and it then gives you the standard pressure, temperature, density, and speed of sound at that altitude. It is neat!

The following graph was generated from data taken from the standard atmosphere. It shows how the air pressure decreases as we ascend to higher altitudes. The altitudes are given in thousands of feet on the horizontal axis, and the pressures are in inches of mercury on the vertical axis.

Click to Enlarge

Here is a table that gives the atmospheric pressure at various altitudes. The altitude is given in feet and the pressure is in inches of mercury.

Altitude           Pressure           Altitude          Pressure
    0,000                 29.92              20,000               13.75
    1,000                 28.86              25,000               11.10
    2,000                 27.82              30,000               8.886
    3,000                 26.82              35.000               7.041
    4,000                 25.84              40,000               5.538
    5,000                 24.89              45,000               4.355
  10,000                 20.58              50,000               3.425
  15,000                 16.88              60,000               2.118
  18,000 *               14.94            100,000               0.329
* This is almost exactly one-half the sea-level value.
      To convert in/Hg to psi, multiply by 0.491.

It is interesting to note that the pressure drops to one-half its sea-level value at about 18,000 feet. The implication is that one-half of all the mass of the atmosphere lies below this altitude. Further, almost (but not quite) a third of the total lies below 10,000 feet. On the other hand, more than one-fifth of the total lies above 35,000 feet.

Pressure lapse rate

As we go to higher altitudes or elevations, the barometric pressure drops. However, the rate at which it drops is not constant; it drops less per thousand feet at higher altitudes.

From data taken from the standard atmosphere, we can determine the average decrease in pressure per 1,000 feet in various altitude ranges, and conversely, the change in altitude required to produce a change in pressure of 1 in/Hg. Here's the result:

                                      Average decrease
      Altitude Range           per 1,000 feet         Feet per in/Hg
  Sea level to 5,000 ft         1.006 in/Hg                     994
       5,000 to 10,000                  0.862                       1,160
     10,000 to 15,000                  0.740                       1,350
Sea Level to 10,000 ft             0.934                       1,070

At atlitudes below 5,000 feet, you won't be in error too much is you simply say 1 in/Hg per 1,000 feet, or, 1,000 feet for each change in pressure of 1 in/Hg. Or even, 10 feet for each 0.01 change in pressure.

6. Lows and highs; Barometric pressure variations from day to day

At first thought, you might expect the air of the atmosphere to distribute itself uniformly over the surface of the earth so that the barometric pressure at the same elevation would be the same everywhere. After all, the water in a pond or lake "seeks its own level," and, except for the tides, the water in the oceans does as well.

But this is not the case with the atmosphere. Several factors, among them the rotation of the earth and the uneven heating of the earth's surface by the sun, come together to cause the air to seemingly slosh about so that at some places it is piled up deeper than at other places. The entire surface of the earth is covered with these mountains and valleys of air, in an ever-changing pattern.

Click to Enlarge

Where the air is heaped up into a pile, the barometric pressure on the ground will be greater than the average value. This forms what meteorologists call a High, meaning a high pressure area. And where the air is less deep, referring to a valley, the barometric pressure will be lower than the average value and will represent a Low, or low pressure region. You have heard the weather people talk about a "high pressure ridge" or a "low pressure trough."

As an experiment, place a pile of water in the middle of the kitchen table and watch what happens. Water doesn't tend to stay piled up! Neither does air. The air on top of the mountains tends to flow down the slopes toward the valleys (referring to the mountains and valleys of air as opposed to actual mountains and valleys). That is, the tendency is for air to flow from a high pressure region toward a low pressure region.

But the rotation of the earth, although gentle and steady, exerts a major influence on the direction in which the air moves. Rather than for the air to move (or, wind to blow) directly away from a high, it will tend to circulate around the high in a clockwise direction with only a small component of its motion being directed outward from the high. And where the air flows into a low pressure region, it will tend to circulate in a counter-clockwise pattern, with only a small inward component.

[Note: the direction of air circulation around highs and lows is reversed in the southern hemisphere.]

To a remarkable extent, the winds tend to blow more or less parallel to the "contours of equal pressure" (isobars) drawn on a weathermap. Typically the angular difference in direction between the isobars and the actual wind is less than 20 degrees, angled out from a high or inward toward a low.

Barometric pressure variations from day to day

It is not realistic to expect the mountains and valleys of air to remain constantly over the same portion of the earth's surface; they move. We see this on the weathermap as the incessant motion of the highs and the lows.

When a mountain of air happens to drift over our little airport, we experience a high barometric pressure. When a valley floats over us, we get a low barometric pressure. It's just that simple.

Altimeter in a WWII P-51 Mustang Click to Enlarge

The range of variation is not all that great, generally 0.5 in/Hg or less above or below the average. The pressure in Honolulu is one of the least variable; the record low is 29.34 recorded in 1936 and the record high is 30.32 recorded in 1905. That's just slightly less than an inch of mercury between the lowest and highest readings. I don't know the highest barometric pressure ever recorded, but a high of 30.50 in/Hg is noteworthy. Here in North Carolina, USA, a high of 30.40 is reason to call home, just because it's rather unusual. Today, at the airport (EXX, 10/25/03), I photographed an altimeter in a P-51 whose Kollsman window showed 30.39. Checked it against the local weather service, and they were reporting 30.36. All these are corrected to sea level, of course.

Kollsman window; Reading = 30.39

The lowest pressures are probably associated with hurricanes. Hurrican Mitch in 1998 produced a low of 27.08 in/Hg, and Floyd in 1999 gave us a low of 27.32. The lowest sea level pressure ever recorded in the western hemisphere was associated with Hurricane Gilbert in 1988. An NOAA aircraft recorded a low pressure of 26.22 in/Hg on September 13, 1988. These are the extremes, however, Normally, lows are not nearly that pronounced, seldom reaching a pressure as low as 29.00.

Pressure Equivalents:

1 Atmosphere (as a unit of pressure) = 14.7 psi = 34 ft water column = 76 cm/Hg
     = 29.92 in/Hg = 1.013 bar = 1,013 mbar
1 bar = 1,000 mbar = 14.5 psi = 29.53 in/Hg = 0.9869 atm
1 psi = 27.68 inches water column = 2.036 in/Hg = 6.859 kPa
1 kPa = 1,000 Pascals = 0.1458 psi

A miscellaneous tidbit, at no extra charge:

One gallon of water weighs 8.34 pounds. One gallon of gasoline weighs about 6 pounds.

1 psi corresponds to 27.68 inches water column or about 37 inches of a column of gasoline.

You will lose roughly 1 psi for every 3 feet of elevation your fuel line rises above the fuel pump. Or, if you have gravity feed, a drop of about 3 feet is required to produce a pressure of 1 psi.

Disclaimer: These figures for gasoline are only approximate because I don't have an accurate value for the specific gravity of gasoline. According to a US Dept of Energy document, a gallon of gasoline may weigh anywhere from 5.8 to 6.5 pounds. I suppose it depends upon the ethanol content, if any, and on the amount of lead it contains, if any

Doc Green